Supervillain Career Fair

Historically villains are not very good at what they do. They usually fail, thankfully. If they were better at their jobs we'd all be robbed blind and walking around with obedience collars on our necks. While some may enjoy that sort of thing, I am vehemently against it.

In general, supervillains are very bad at their jobs. Which leads me to believe that they should change occupations.

Lets talk about Calendar Man. He's a Batman villain who commits crimes on specific days of the year. It's kind of his gimmick. But committing crimes on specific days doesn't make you more successful. It actually hinders you quite a bit, allowing Batman to punch you repeatedly in the face. This is a bad thing. Unfortunately, his gimmick was stolen by the Holiday Killer in Batman: The Long Halloween. That means that Calendar Man is not only ineffective, he's not unique.

Now let's consider an old favorite: Spider-Man's villain, Doctor Octopus. Recently (Amazing Spider-Man #600), it was revealed that years of getting punched in the head by Spider-Man had caused Doc Ock to develop neurological damage. In other words, his head got punched so much his brain began to turn to mush. Doc Ock wrapped himself up like a desicated mummy and tried to get revenge on NY one last time only to (you know where this is going) get punched by Spider-Man. If there is someone who needs to pursue a new line of work more, I do not know him.

Then lets examine some villains who did change occupations. The Riddler went from being an insane criminal quasi-genius to a private detective. This change in occupation netted him more money and respect than he ever received as a criminal. It's also been over a year since he swallowed his own teeth because Batman punched them in. This is definitely a step in the right direction. Norman Osborn went from wearing a form fitting green and purple costume throwing pumpkin-shaped explosives from a bat-glider to becoming America's top ranking national security officer. And that's worked pretty well for him too. He now lives in a beautiful penthouse and gets to desicrate Iron Man's armor on a daily basis. Of course things may change in January during Siege (I do love's me some classic Thor beatdowns).

Regardless, villains who have changed occupation from traditional super-villainy to something else have seen a dramatic increase in prosperity. And that's why we need a supervillain career fair. In fact, if they were smart, superheroes could get together and organize this fair. Batman and Superman should work to get their rogues connected with major companies in order to make everyone's lives easier. Mr. Freeze would be better off working for Frigidaire. Poison Ivy could work for Greenpeace. The Toyman could work for Hasbro. Clock King could improve the design of a Rolex tenfold. I'm actually pretty sure Braniac already works for Apple.

It's so ridiculously obvious, I'm really surprised that no hero has tried it before. But they should.

I mean shouldn't it be every hero's goal to turn this:


...into this?

share on: del.icio.us digg reditt su

Life Insurance Now a Moot Point

In light of the recent trend in comics events (namely Blackest Night and Necrosha) it seems like no comic book character can stay dead. While this has always been true, now more than ever, the dead are suddenly seeming very lively.

Now, we've talked about insurance a lot in the past. Namely here, here, here, and oh yeah here. We like the topic. Mostly because an industry based on risk assessment in a world where aliens invade every Friday is too ludicrous not to talk about. And if there's one thing we like at Ecocomics, its ludicrous stuff.

But life insurance is another interesting point to deal with. Lets use someone as an example who seems like they're going to live a nice stable life. Let's say we use Jean Grey in the early 80s. So Jean has nice responsible parents who love her deeply. They don't want to think that its possible that their daughter could die, but like responsible adults, they need to plan for contingencies. Especially when their daughter is the kind of person who picks fights with the Living Monolith. So the Greys purchase a plan for their daughter. Then when she gets exposed to solar radiation, possessed by an alien life force, and blasted with a laser on the moon, it's time to collect on their policy.

The Greys then use the money from their daughter's life insurance policy to give their daughter a nice respectable funeral where all of her friends (including the blue elf creature, hairy canadian, and russian metal guy) and her laser blasting fiancee can mourn her properly.

And then the Fantastic Four finds Jean in a cocoon at the bottom of the Hudson River. What happens now? Has fraud been committed? Does the insurance company who paid out for Jean's death get their money back? If so, who pays them?

And this is the confusion that results from only one death. What happens when every mutant in Genosha comes back to life? What happens when every dead person in the universe comes back with a black lantern ring? Granted in both of these cases, people in insurance companies are likely too busy trying to keep the reanimated corpses from devouring their hearts to think about the finer points of this debate. But the debate remains. Theres a lot of folks re-animating and a lot of insurance claims which would seem to now be invalid.

share on: del.icio.us digg reditt su

The Bennett Bailout

Amazing Spider-Man #612 by Mark Waid and Paul Azaceta (2009)

The latest tale in Spider-Man's never-ending struggle demonstrates just how powerful the economy is as both an instigator of public discontent and as a motivation for supervillains. If we think about it, many supervillains form because they are either down on their luck or run into some sort of trouble with their finances. As Ezra Klein noted, the Sandman turned to a life of crime in Spider-Man 3 because he was unable to afford medical treatment for his daughter. Most ordinary thugs you see in comics (and a fairly large amount of brand-name villains too) commit crimes that are financially motivated. Even the Joker, depending on your interpretation has origins stemming from financial peril. A struggling comedian trying to support his family, he pulls a heist at a chemical plant to make some cash and...well, you know the rest.

Obviously the state of the economy is important here. We've discussed before how it's possible that an economic recession could lead to more crime. This applies mostly to your orindary street-thug types (remember this guy?), but if left unchecked we know that these low-tier criminals could escalate to be formidable opponents.

But now to top it off, we have a new way that the economy can affect the state of superheroics: public opinion.

Dexter Bennett, former construction tycoon turned newspaper owner, has just engineered the first official federal government bailout of the newspaper industry (specifically, his own newspaper The DB). If you think that this is ludicrous, recall that back in September President Obama hinted at doing something like this. To be fair, Obama was mainly looking at proposals to give extra tax breaks to struggling newspapers if they agreed to restructure as nonprofits. Waid doesn't go into much detail in Amazing Spider-Man, but it looks like what's happening is that the feds are just throwing money at The DB with no expectation of restructuring, public ownership, or...anything. This sort of makes you think about what the federal government is getting out of keeping The DB alive. Or how exactly Bennett pulled it off in the first place.

However, this is all besides the point. Far more interesting is the unintended result of this "Bennett Bailout." Remember the classic Spidey villain, Electro? The one who can...electro-ize stuff? The one we haven't seen in a while? I wonder how he can the federal government's actions to his advantage:

You see, NYC isn't exactly happy with the Bennett Bailout. Citizens feel that their taxpayers' money is being wasted to support greedy, capitalist fat cats sitting in their mansions as they suck the life out of the middle class. If anyone has read the supervillain instruction manual, they know that praying on the fears of the public is by far the easiest way to attack a superhero. And what do people fear more than anything else in the world right now? It's not a crazy former villain who shoots electricity out of his eyes. It's Wall Street.

Interestingly, this is precisely what J. Jonah Jameson has been trying to accomplish for years. By writing scathing critiques of the webcrawler in the former Daily Bugle, he was trying to instill a permanent sense of fear. He argued that Spider-Man increased crime. He argued that Spider-Man was a danger to society. He tried everything and only marginally impacted the public's perception of Spider-Man.

In one day, Electro managed to change all of that. He managed to completely change his own reputation from being a terrorist to being a servant of the labor force. He also managed to persuade the public that Spider-Man was part of the capitalist conspiracy. AND he did it without any intention of involving Spider-Man at all! Turns out Electro had lost the fortune he had in various investments when the economy went sour. He's out primarily for personal gain.

What's the difference between Electro and Jameson? Jameson never thought to use the great instigator of fear. The...economy!


The lesson here is that if superheroes want to be thorough, they better start getting their PhDs and involving themselves in public policy.

share on: del.icio.us digg reditt su

Sorry for the Sporadic Posting

Irredeemable #7 by Mark Waid and Peter Krause (2009)

Hey guys--sorry for the irregular post schedule recently. The months of November and December are very busy for us as we have lots of deadlines, etc. We should be back to updating on a daily schedule starting next week (though there will definitely be some posts this week too). To wet your appetite, here are some topics you have to look forward to:

• Government bailout of the newspaper industry
• Someone finally figured out how to use economics against Spider-Man
  
• Indian Casinos
• More on strange deals with the Devil

Thanks for reading!

share on: del.icio.us digg reditt su

Ecocomics Explains: Opportunity Cost

Ecocomics Explains is a new recurring feature of this blog. Each week, we will discuss a different economics concept--ranging from more basic ones to more advanced and mathematically involved ones--and highlight some examples from comic books that reflect the ideas. We will also include a rating system in each post to show the difficulty level of the concepts. 1 Greenspan refers to a very basic concept, 2 Greenspans refers to a more intermediate concept and 3 Greenspans refers to an advanced concept.

(1 Greenspan)




Opportunity cost is a fairly basic economics concept. Anyone who has taken any introductory microeconomics course certainly knows about it. It is usually one of the first topics introduced in class. You're probably familiar with a number of classic examples, such as having a choice between hamburgers and pizza or guns and butter. Those of you who have never taken economics before are still likely familiar with the intuition behind it, but maybe not know the terminology.

Opportunity cost stems from the fact that resources are scarce. In fact, the entire field of economics is basically the study of how individuals and societies allocate scarce resources. When a society chooses to place greater emphasis on the production of one good, then production of another good necessarily has to decrease. Similarly, consumers who have a fixed income have to make choices between which goods to buy. Purchasing more of one means purchasing less of another. The opportunity cost of a good, then, is what an individual, firm or society gives up in order to have one particular good. It is the value of the highest valued foregone alternative (this is the definition used in the third edition of Microeconomics Michael L. Katz).

This doesn't just apply to production and consumption of goods, however. It embodies the idea of tradeoffs, which is something that we all experience on a daily basis. And it happens in comic books all the time too!

To see how this works, let's consider the case of Spider-Man. Spidey is a fascinating study because basically the entire point of his ongoing series is to highlight his struggle to maintain a balance between his personal life and his obligations as a superhero. Every day for Spider-Man is an exercise in opportunity costs.

The Amazing Spider Man #600 by Dan Slott and John Romita Jr. (2009)

See here how Peter Parker is making a choice between earning some more money to support his crime-fighting double life and attending Aunt May's rehearsal dinner (she was recently married to J. Jonah Jameson senior). Let's look at this example in a bit more detail, but spice it up a bit. Suppose that Peter has 60 minutes (1 hour) of free time. In that free time, he can either go out and fight some thugs on the street or he can choose to attend Aunt May's rehearsal dinner and spend time with his family. Also, let's say that it takes Spider-Man 12 minutes to take down an ordinary street thug and that it takes 6 minutes with his family to earn him a "brownie point." This means that Spider-Man has a production equation of the form:

12x + 6y = 60

where x is the number of criminals Spider-Man takes down and y is the number of brownie points he earns at the May residence. Given this equation, if Spidey decides to take down 3 thugs (x=3), then we have:

12(3) + 6y = 60
36 + 6y = 60
6y = 24
y = 4

Thus, if Spider-Man spent his hour taking down 3 thugs, he could have also had the time to earn 4 brownie points.

This can be represented graphically as follows:

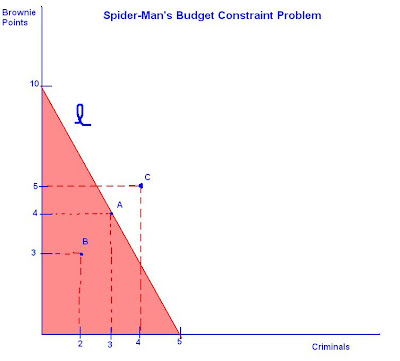Note: Not Drawn to Scale. Not drawn particularly well either. By now you've noticed, I prefer drawing my graphs in MS Paint. Lost art, really.

In the graph above, line "l" represents Spider-Man's "budget constraint." This is just a visual representation of the bundles of goods that our webcrawler can "afford" with his given "income." In this case, income refers to Spider-Man's allotted time schedule, the goods are brownie points and criminals put in jail, and costs refers to time in minutes. Any point on the graph beneath line "l" is in Spider-Man's "feasible set." This is the set of all combinations of criminals and brownie points that Spider-Man can possibly afford in his hour of free time. Any point that is in the pink shaded area of the graph is feasible.

Take our example above. If Spider-Man chooses to fight 3 thugs, which would take 36 minutes, he could then only earn 4 brownie points. This is reflected as point A on the graph. Notice that point A is exactly on the budget line. Hence, Spider-Man is using all of his time towards one of the two goods. This is an efficient use of his time. Suppose instead that Spider-Man decided to fight 2 criminals and earn 3 brownie points (point B of the graph). In minutes, the bundle would cost:

12(2) + 6(3) = 24 + 18 = 42 minutes.

Point B, although being in the feasible set, is not efficient. The reason is that Spider-Man is only using 42 minutes of his time, which means he has 18 minutes left over that are not being devoted to one of the two goods that exists in this universe. With that 18 minutes, he could be fighting more criminals or earning more brownie points. But he isn't.

Now consider point C of the graph. At this point, Spider-Man fights 4 thugs and earns 5 brownie points. In minutes, this bundle would cost:

12(4) + 6(5) = 48 + 30 = 78 minutes.

Obviously, Spider-Man only has 60 minutes and therefore cannot purchase this bundle of goods. Point C is therefore not feasible.

If Spidey chose not to attend Aunt May's dinner at all, but instead to spend the entire hour fighting crime, he would be able to bring down a maximum of 5 street thugs in the 60 minutes. If he chose to sacrifice his hero duties for an hour and spend its entirety with the family, he would be able to earn a maximum of 10 brownie points. These points are the x and y intercepts of the graph and are the endpoints of the budget constraint.

So, where is Spider-Man's opportunity cost in this graph? It's actually the slope of the budget line! Notice that the slope is -2. This represents the opportunity cost of one good in terms of the other. So, the opportunity cost of one criminal is 2 brownie points. To put one more criminal to justice, Spider-Man would have to sacrifice 2 brownie points that he would have otherwise gained by being with his family. Conversely, to gain two more brownie points, Spider-Man would have to sacrifice fighting 1 criminal.

Those are the basics of opportunity cost in a nutshell. I even threw in a little bit of linear budget constraints. Once we discuss utility maximization, we can bring in other factors. For example, we all know that Spider-Man suffers from immense guilt over the death of Uncle Ben and would likely derive more utility from fighting a criminal than maintaining his personal life. We can factor all (or most) of this in to an optimization problem. But this is a post for another time.

share on: del.icio.us digg reditt su

Is Class Warfare Inevitable?

While reading a discount copy of "Strange Killings" featuring the the Warren Ellis creation, Sergeant Major William Gravel, I began to wonder about class differences.

You see, Bill Gravel is a magician. But he's a very particular magician, namely a combat magician. His skills to manipulate reality (including warping the path of bullets, visual illusions, and summoning demonic horses) make him an extremely effective killing machine. This allows him to succeed both as a private mercenary and a soldier in Britain's SAS. His abilities are even strong enough to grant him access to two societies of the most powerful magicians in Great Britain, namely the Minor Seven and the Major Seven. But despite his amazing abilities, William Gravel considers himself a blue-collar man. His main goal is to protect his country, accrue enough money to pay for his drinking habit, and kill a few wankers along the way. Modest goals to say the least.

In the first arc of Warren Ellis's ongoing "Gravel", Bill's meager life goals cause him some trouble. He is thrown out of the Minor Seven by the six other members of the group and replaced by a paranormal archaelogist who has a more aristocratic bent. The main reason for removing Gravel from the Minor Seven is very simple and very elitist: he's a peasant. Gravel uses his magical powers for meager ends and doesn't behave as is expected for one of the most powerful magicians in Britain.

Gravel's response? He kills the bastards, one by one. In fact the first arc of "Gravel" is just the systematic murder of the men and women who consider themselves to be Gravel's betters.

The point of this being that there is class warfare in even such a small bizarre subset of the world. If even the Magicians of Great Britain can be torn asunder by social and economic disparity, is there any hope for the rest of world?

share on: del.icio.us digg reditt su

Reading List for the Week Of 12/2/09

Mark and I thought it would be fun to start a weekly post where we recommend the best of our reading lists. If you guys are reading anything we aren't, we would also love for you to comment and tell us about it. We need suggestions for more reading material anyway!

This first post will include last week's comics as well as well as what we're planning on reading this weekend (since we didn't have a chance to read new releases yet).

Starting next week, we'll also include some economics writings too!

Comics From Last Week
Chew #6 by John Layman Rob Guillory
Tony Chu is a cibopath. That means he gets psychic impressions from anything he eats. If he eats a hamburger, he can tell you the origin of the meat. Meanwhile, a bird flu epidemic has caused a nationwide scare prompting the government to expand the regulatory powers of the Food and Drug Administration and ban the wholesale of chicken. See our previous posts on Chew here and here.

Criminal: The Sinners #2 by Ed Brubaker and Sean Phillips
Anyone, comic or non-comic fan, who isn't reading Criminal by now is really missing out. For fans of the pulp, crime-noir genre, this book is absolutely critical. Not to mention, Criminal: The Sinners #1 is a great jumping-on point for new readers. Each arc in the title if relatively self-contained (albeit having minor parallels with other arcs).

Invincible Iron Man #20 by Matt Fraction and Salvador Laroca
This is probably my favorite superhero book out right now. Tony Stark has been ousted from his position at Stark Industries and is on the run from Norman Osborn. It would appear from the last issue that Norman has finally beaten him. But with the start of this new arc, we're in for a treat. Matt Fraction continues to weave an incredibly intricate and layered story that shows Iron Man in a brand new light.

Gotham City Sirens #6 by Paul Dini and Guillem March
If you've ever seen the wildly successful and brilliant Batman: The Animated Series, then you pretty much know what to expect whenever Paul Dini is writing a Batman book. Currently, he has two ongoing titles: Streets of Gotham, which more or less focuses on the daily operations of all facets of Gotham City, and Gotham City Sirens, which focuses on the ladies of the city: Poison Ivy, Catwoman, and Harley Quinn. Nothing too groundbreaking in this issue, but it is a load of fun.

Amazing Spider-Man # 613 by Mark Waid and Paul Azaceta
The best thing about reading Spider-Man is watching Peter Parker balance his superhero responsibilities with his life as a civilian. This issue continues that trend, only now Spider-Man becomes, wait for it, a public menace! Except this time it's because of economics! Sort of. Oh yeah, and Electro becomes a public hero? Very fun things going on in the webcrawler's world this week.

Thor Giant-Size Finale #1 by J. Michael Straczynski and Marko Djurdjevic
A bitter-sweet ending to J. Michael Straczynski's fantastic run on Thor. This is bittersweet because it provides a nice, fun ending to many of the plot threads that Straczynski has developed ove the course of his last 16 issues. On the other hand, it is the end of the best Thor run since Walt Simonson's landmark 4 year run in the mid-80s. In this issue we see some really cool things, including the final fate of the most courageous short order cook in history, William the Warrior.

Uncanny X-Men #517 by Matt Fraction and Greg Land

Matt Fraction's run on the Uncanny X-Men hasn't been socially or politically significant. But goddamit, it's fun. This issue sees the combined forces of the X-Men, Namor, and Magneto battle five Predator X creatures. What is a Predator X, you may ask? It's a creature the size of a monster truck with razor sharp teeth, an nearly impenetrable metal hide and a voracious hunger for mutants. And seeing Namor punch one of these things in the face while dragging it to the bottom of the ocean is truly a blast. And Greg Land's artwork, while occaisionally creepy and quasi-pornogrpahic, is pretty to look at.

Comics This Week
Jonah Hex #50 by Jimmy Palmiotti, Justin Gray, and Darwyn Cooke
Superman: World of New Krypton # 10 by James Robinson, Greg Rucka and Pete Woods
The Marvels Project #4 by Ed Brubaker and Steve Epting

Thor # 604 by Kierron Gillen and Billy Tan
Ultimate Comics Spider-Man #5 by Brian Bendis and David Lafuente
Uncanny X-Men #518 by Matt Fraction and Terry Dodson

Misc. Comics
Daredevil: Born Again by Frank Miller and David Mazzucchelli
Remember when Frank Miller wrote the best comic books in the industry? Remember when he had basically attained "do no wrong" status? Daredevil: Born Again was at the height of this time period. Not quite The Dark Knight Returns, but I would rank it up there with Batman: Year One.

The book tells the story of Matt Murdock's descent into insanity as the Kingpin discovers his secret identity and them systematically ruins his life. It's a story about loss, hope and redemption. If this sounds familiar, it's because Kevin Smith basically wrote the exact same story years later and called it Guardian Devil.

This book is a must read for any Daredevil fans.

share on: del.icio.us digg reditt su

Priorities, India.

Supergod #1 by Warren Ellis and Garrie Gastonny (2009)

It may not be China or Russia or the United States who will be responsible for the world's ultimate destruction, but India! OK, it will probably be the United States, but India seems to be at least partially responsible in Warren Ellis' new series, Supergod.

Ellis hits the nail on the head here regarding India's massive problems with sanitation, overpopulation and water supply. He notes that a thousand Indian children per day died from sickness related to the population in the river Ganges. According to a 2006 World Health Organization fact sheet, about 456,000 Indians died in 2002 due to Diarrhoeal diseases. 20% of the deaths of children under 5 years old were from Diarrhoeal diseases. And apparently a 2003 government report indicated that only 30% of India's waste water was being treated, with the untreated supply flowing into rivers. One other thing: as of 2006, the population with sustainable access to improved drinking water was 86% in rural areas and 96% in urban areas What about access to improved sanitation? Only 52% in urban areas and...18% in rural areas! Yikes!

Of course, these are more recent statistics than the ones I think Ellis is alluding to, since he refers to the post-Cold War period. I don't have those ready (and will certainly appreciate if anyone finds them!), but an interesting bit of information about that period was that it was the setting of economic crisis of 1991. Growing fiscal imbalances had caused the government to nearly default. Then came economic liberalization! The country opened itself up to foreign direct investment, reformed capital markets, and privatized/decentralized domestic businesses. GDP grew and many people eventually escaped poverty.

However, regulation of the water and sanitation infrastructure was delegated to local municipalities. These institutions are unfortunately very weak and don't exactly have the resources to manage the upkeep. And so many people continue to get sick and remain without access to adequate water supply and proper toilets.

All this and India still finds the money (and this is before the full benefits of economic liberalization were realized, mind you) to fund a team of scientists to create its own superhuman. More than a superhuman...a Supergod! One who decides that the only way to save India is to, well...


Priorities, India. Priorities.

share on: del.icio.us digg reditt su

Doctor Strange's Health Care Promo

A few weeks ago, we asked readers their thoughts on who would be the best superhero spokesperson for health insurance. Congratulations to our winner, Will, who came up with a clever promo/commercial for our favorite hero physician (next to Dr. McNinja), Dr. Strange! Here is the answer:

Dr. Strange brings the unique perspective of a man who was a doctor (leading neurosurgeon), a patient with a pre-existing condition that affects his employment options, and, currently, lost his job as Master of the Mystic Arts. I have no idea what health plan the Master of the Mystic Arts has, but he had access to a great deal of resources now not available. Here is how I see his ad going: "Hi, my name is Stephen Strange. To many, I am known as Dr. Strange, Master of the Mystic Arts. Unfortunately, like so many Americans I have recently lost my job as Master of the Mystic Arts and, in the process, I have lost my health insurance. With my numerous enemies, injuries, possessions, and other hazards, my premiums are far too high for me to afford and I am left uninsured. As a former neurosurgeon, I know the cost to the hospitals and the medical system of uninsured like me and that cost is also felt by you. Please join me in trying to end this broken system and, by the Hoary Hosts of Hoggoth, we can have an affordable public option that insures all Americans."

This is a wonderful and hilarious response. The great thing about Dr. Strange as a spokesperson for health care reform is not only that he has seen the perils of our health insurance system from within, but that he would also be a terrific proponent of reforming areas of health that have not received enough media attention, namely health care delivery and public health. Furthermore, Dr. Strange has gained so much prominence (despite not having his own ongoing title and not being a registered superhero) that I would consider him among the A-listers in the superhero business. Finally, the commercial that was Will envisions appeals to the public's sympathies by telling a story that many people can relate to (well, inasmuch as they can relate to someone with powers of the dark arts). The combination of all these factors is likely to garner considerable support for the campaign.

Again, thank you all for your wonderful comments. Will, please email us at ecocomics dot blog at gmail dot com with your top five comic choices and address.

share on: del.icio.us digg reditt su